On Friday I attended a CPD session ran by Dominic P. Tremblay entitled ‘Everything is Awesome with LEGO® Math!’ as part of the Practical Pedagogies conference organised at our school. As you can imagine the draw of playing with LEGO whilst thinking about mathematics was huge and the room was packed with educators eager to get their hands on those little colourful blocks. Dominic encourages students to use creativity to represent numbers, fractions, multiples and patterns. For example, the following construction is a visual representation of the number three. Can you see why?

The session was pitched mainly at primary mathematics reflecting the participants of the workshop, as the pictogram that we represented of ourselves opposite demonstrates (note small group of secondary teachers highlighted). In his keynote presentation at the start of the conference, Ewan Macintosh spoke of the need for provocation in learning. Touching, manipulating and constructing the blocks for the tasks we were given was enough for me to get a bucket-load of ideas of how I could use LEGO for secondary maths.

In this blog post, I will summarize some of the ideas I had during the session for how I might use LEGO blocks in the classroom. I hope that the list might grow in time.

1. Blocks could be used for data handling in pictographs or barcharts (see above).

2. Visualising Fractions. Create a fraction to represent 2/3, 5/8, …These questions could be simple or more complicated compositions, as the shapes below demonstrate. These could then be attached to a number line.

3. Flowers could be used to represent powers (exponents) since stems can be added above and below. Here's my attempt to represent . The total number of flowers could represent the geometric series 3 + 3² +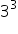

4. Create a sequence of shapes that follow a geometric pattern.

5. Gears could be used to represent ratios or even rates of change.

6. Make a reflection of a shape given to me by a partner. We could do some simple 2D reflections, but why stop there!

7. As above for rotations.

8. Bricks could be used to represent positions in a coordinate grid. A cooperative game could be played where player 2 tries to recreate the same grid as player 1 from descriptions of positions. Again, why stop at 2D coordinates?

9. Vectors could describe movement from one position on the grid to another.

10. Use blocks to represent volume factor of enlargement.

I really enjoyed Dominic's session and I left feeling that there was a lot of potential for using LEGO blocks in the classroom. The next stage is now to build up a large stock of LEGO blocks for the mathematics department. I thought that I might be able to get some cheap secondhand blocks or ask for donations from families of the students (surely there must be some teenagers who would be ready to part with their LEGO blocks!). Online manipulatives of LEGO blocks exist, but I don't think are as good as the 'real' thing. A google chrome add-in is available and LEGO Digital Designer can be downloaded for free. If you want to find out more about Dominic's work with LEGO visit his Facebook page.