InThinking Subject Sites

Subscription websites for Cambridge IGCSE teachers & their classes

Find out more


Sitemap
Recommendations
Other subjects
Brochure
Student access tools
 You need to log-in or subscribe in order to use Student access.

Circle Circumference

'Find out all about circles with this investigation. '

What shape has no starting point, no ending point, a single centre and no straight sides?  Actually, you might like to argue that it has an infinite amount of straight sides!  The circle is such an important shape to us: just think how difficut it would be to ride your bicycle if we hadn't invented the circle.  In this investigation you are going to discover an extremely important relationship about circles.  You'll need to make some accurate measurement and be careful not to make assumptions.

Resources

  • You will need a large sheet of blank paper, a ruler, a pair of compasses and some string.
  • For the second part of the investigation you will need access to a computer with internet access 

Practical

1. With a pair of compasses, draw 3 different circles each with a different radius. 

 

2. Using a piece of string, carefully measure the distance around each circle (the circumference).

3. Record the length of the diameter and the circumference of each circle in the table below.  You can also record the results of a partner here too.

4. In the 3rd column work out circumference divided by diameter for each circle.
    Clue: for the first circle, type the following in cell C2: =B2/A2

5. What do you notice about these results?

Nearly Three

  1. It is a bit tricky to measure the distance around the circle, especially with a piece of string.  Can you think about some possible sources of error in your measurements.  Discuss with a partner.

  2. You might think from your results that the circumference is three longer than the diameter.  The following applet will help you decide of that is true or not.  The applet shows a circular piece of liquorice being unrolled. Drag the slider to unroll the circle and find the distance around the circle. Compare the distance around the circle with the diameter. 

Notice that it is not exactly 3 times bigger.You can change the diameter of the circle by entering different amounts in the input box.

More Accuracy

So you should have seen that the circumference is a bit more than three times the length of the diameter.  We need to be able to make more accurate measurements of the diameter and the circumference than you can manage with your ruler and a bit of string.  You can construct a circle using Geogebra and get very accurate measurements (see help video below if you require assistance with geogebra): 

  1. From here you can open a new blank Geogebra file.  
  2. Construct a circle. 
  3. Measure the radius.
  4. Measure the circumference.
  5. Make the calculation of circumference divided by diameter, as before.  
  6. Change the dimensions of the circle and see what happens.
  7. How many times bigger is the circumference than the circle?

 Geogebra Help Video

Summary

You should now have discovered the relationship between the diameter and the circumference of the circle.

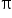The number of times bigger is 3.14159265...  The dots here mean that the number is not finished! In mathematics, we give this special number a name, pi with the symbol straight pi.  If you want to learn more about this mysterious number, you might like to try another investigation  Discovering Pi

Share:    

All materials on this website are for the exclusive use of teachers and students at subscribing schools for the period of their subscription. Any unauthorised copying or posting of materials on other websites is an infringement of our copyright and could result in your account being blocked and legal action being taken against you.