Congruent halves and transfomations

These problems are brilliant and have been around for ages. You need some real lateral thought to figure out how to divide the shapes in two such that both of the halves are congruent shapes! What is different about this activity, is that you are being asked not only to solve this bit of the problem, but to construct these shapes using technology and by drawing half of the shape and transforming it to create the other half! This will involve reflection, rotation, translation and maybe some combinations of the three. You will need to create and use mirror lines, centres of rotation and translation vectors and get the size and positions correct. You will know when you have done it because your two shapes together will make the original shape you staretd with. See the eample below...
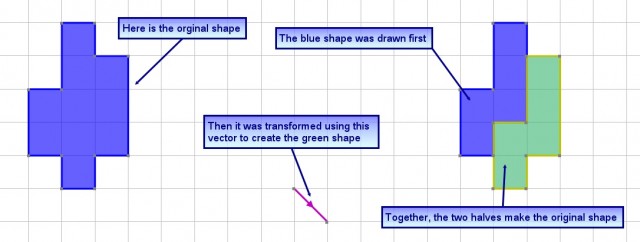
and see more examples of how to do it in this video that explains and outlines the task. (This screen cast was made using autograph software, but it could easily be done in any dynamic geometry software)
Resources
This activity can run right from this page, but it does sometimes help to have the ![]() Congruent halves problems on a bit of paper too!
Congruent halves problems on a bit of paper too!
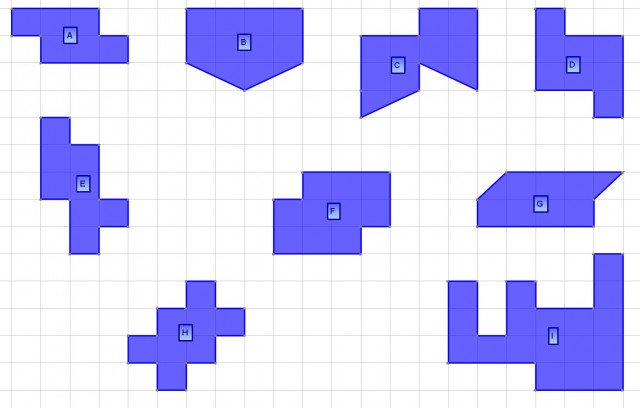
Target shapes
Here are the target shapes!

Help videos
The following videos are designed to help students use the software. They are made using autograph software, but the exercise is easily transferable to other dynamic geometry software. (As I make help files for geogebra I will add them)
Adidng points, drawing shapes etc.


Reflections


Rotations


Translations


Description
- Start with an example like the one above (at the top of the page) and try to split the shape into congruent halves.
- Recognise that the idea is to draw the shapes by first drawing one of the congruent halves and then transforming it to make the other.
- The transformation needs to put the second half in the correct position such that the combintaion of the two halves makes the original shape.
- Try all the shapes on offer, either by looking at this page or the printed worksheet.
- You will need reflections, rotations and transformations and, in each case, you will need to think carefully about the position of mirror lines, centre of rotation and/or the size of translation vectors.
- Think about multiple ways of making the same shape.
- Think about making some new and challenging shapes yourself.
Teacher Notes
This is just a quick summary of why I think this is such a rich classroom activity. I am often inspired by the notion that 'Opportunties to practise can be found inside rich tasks'. This is a great example of that for me!
- In the beginning there is an element of puzzle solving that is engaging. From my experience, students believe they can separate the shapes according to the rules and want to have a go.
- As the exercise progresses, it becomes apparent that in some cases there is more than one solution and that in others, the solution requires a bit of lateral thought.
- It is one thing to find the solution with pencil and paper, it then becomes entirely another thing to reproduce the shapes with transformations, thus taking the challenge on to another level.
- Finding centres of rotation is probably the trickiest element and this exercise really helps students to think about and explore this idea.
- Otherwise, there is plenty of practice of transforming shapes involved, along with a good bit of reverse engineering!
