Teacher Notes

A great triangle mystery! These 24 triangles can be split in to 8 groups of 3 similar triangles by matching the angles. Once in groups students can use proportional reasoning to work out the missing lengths on the similar triangles. This is one of those activities that runs itself. Present the triangles and say 'Find out the missing lengths' or add some structure depending on the class. The activity can be extended in to Pythagoras's theorem and trigonometry. A great set of classroom props!

Resources

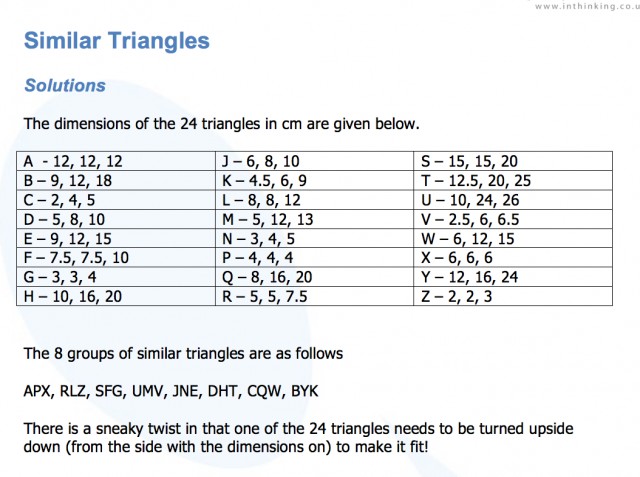
• Each group requires a pack of 24 triangles. Within this pack there are eight sets of three similar triangles. Three are scalene with no right angle, two are scalene with a right angle, two are isosceles with no right angle and the last set are equilateral. The triangles will print to scale but it is not necessary to tell students this.
• There is also an activity sheet for students on which to record the results of any work they do. 
• Here are the  Similar triangles solutions. A screen shot is also shown below.

Activity

There are a variety of possible approaches for using these triangles, each of the following represents a possible starting point but they could also follow each other as activities. The most open way to run the activity is to give out the triangles and ask students to deduce the missing lengths without using a protractor or a ruler. Structure can be added as the teacher sees fit for their class, but I would recommend that in the first instance the most open approach is tried. The key point for students is to recognise that some of the triangles have the same angles as each other. They can establish this by placing the angles on top of each other, without measuring.

• With the class divided into groups, each group is given a set of triangles and asked to classify them in any way they see fit. Having done this, groups are asked to share the process they went through. One way of doing this is to ask each group to leave one person with their work to explain while the others go around the room looking at the others. Alternatively, thoughts can be collected by the teacher on the board. Students might then be asked to consider an alternative classification.
• Can the triangles be classified into scalene, isosceles and equilateral? This is is left as a question because it depends very much on the reasoning students use without access to the missing lengths or rulers and protractors. For example they might conclude that a triangle is isosceles because it has a line of symmetry. It may also be appropriate to ask whether or not equilateral triangles are a subset of isosceles triangles.
• Students should be challenged to ask how they know.
• Are any of the triangles enlargements of eachother? Classify the triangles by this means. (ie all triangles in a given group are enlargements of each other. There is potential for interesting general discussion with this task – ‘How can we tell if they are enlargements?’ The triangles can be placed on top of each other in any of the three shared angles for a visual demonstration.

Having established the sets of similar triangles

• What are the scale factors from one shape to the next? Leading to statements such as ‘the scale factor from shape A to B is …..’ How do you know? For each set of three there are six such statements that can be made.
• What are the missing lengths? How do you calculate these? For each set of triangles there are 4 missing lengths. Each can be calculated in different ways. This question may allow students to use their intuition to solve problems involving similar triangles and, as such, use already existing knowledge to solve the more difficult ‘looking’ problems. The extent and nature of the justification students provide for their answers is flexible and depends on the varying objectives of the teacher.

Extensions

As suggested, these can be really useful props to extend in to different directions, either at the same time or at a later date. Below are some examples.

• Use Pythagoras’s theorem to establish which of the triangles have right angles.
• What are the areas of these triangles? 
• Investigate the area scale factors between these triangles.
• Calculate all the angles in the other triangles using the cosine rule and sine rule.
• Now work out the area of these triangles as well.
• Now investigate the area scale factors between these triangles as well.

Solutions