Surround TN
Teacher Notes
This is an excellent investigation to get students to describe the pattern generated by surrounding a shape with squares. They should be able to find a formula and link the formula to what they see. The activity is well structured, but it could be set in a far more open-ended way.
The investigation requires students to be systematic in their approach. A much more guided approach could be given to students; however, this would very much detract from the task itself. The formulae are provided below, but hopefully teachers will want to try the investigation for themselves before they give it to their students!
Challenge 1
Surrounding a 1 by 1 square
| Layer | Squares |
|---|---|
| 1 | 8 |
| 2 | 16 |
| 3 | 24 |
| n | 8n |
Challenge 2
Surrounding an x by x square
| Size of Square |
Squares |
| 1 | 8n |
| 2 | 8n + 4 |
| 3 | 8n + 8 |
| x | 8n + 4x - 4 |
If students are able to find the nth term of an arithmetic (linear) sequence then they should be able to find this general formula for the square. Students may even be encouraged to adopt a graphical approach:
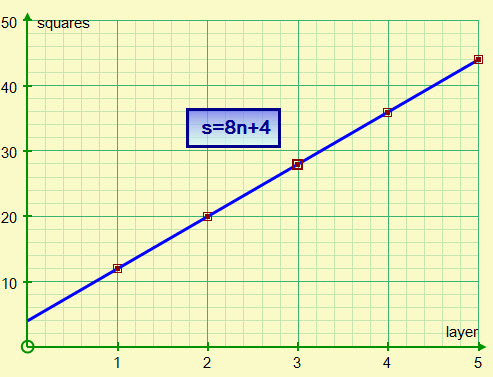
Image courtesy of Autograph
Challenge 3
Surrounding an x by y rectangle
It is extremely difficult to make further progress without considering carefully what the formula means. It is possible to consider rectangles with dimensions x by 1, x by 2, x by 3, etc and bring these results together to find a formula for an x by y rectangle. However, a far more elegant solution is to think carefully about what is happening when we add each layer, i.e. that the width and the length of the rectangle increases by two (a clue to this was given in the student conversation on the main activity page). A possible approach is given below:
| Layer | Squares |
| 1 | 2(x + y) + 4 |
| 2 |
2(x + 2 + y + 2) + 4 2x + 2y + 8 + 4 |
| 3 |
2(x + 2 + 2 + y + 2 + 2) + 4 2x + 2y + 16 + 4 |
| n | 2x + 2y + 8(n - 1) + 4 |
Challenge 4
Surrounding an x by x by x cube and an x by y by z cuboid.
This is a really challenging extension problem!