Notes
Teachers Notes - OXO
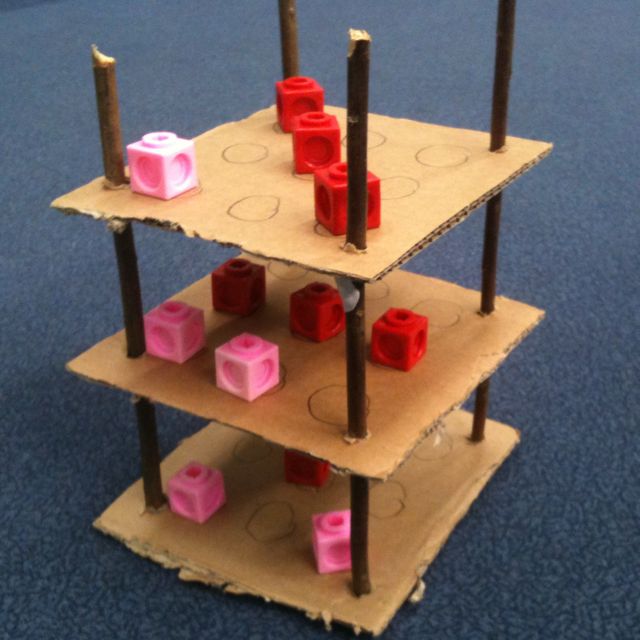 This can be a very rich source of mathematical activity on a number of levels and is one of my favourite things to do with a class. The activity offers great examples of real situations that lead to algebraic sequences. It gives students a great opportunity to test their powers of intuition in an enjoyable and engaging context. It is also a great example of a situation where the more complicated context can be the most instantly engaging! Counting the number of possibilities to get three in a row in a 3D game invariably causes thought, discussion, and debate and brings some fabulous points out.
This can be a very rich source of mathematical activity on a number of levels and is one of my favourite things to do with a class. The activity offers great examples of real situations that lead to algebraic sequences. It gives students a great opportunity to test their powers of intuition in an enjoyable and engaging context. It is also a great example of a situation where the more complicated context can be the most instantly engaging! Counting the number of possibilities to get three in a row in a 3D game invariably causes thought, discussion, and debate and brings some fabulous points out.
How
The following is some practical advice about how the activity might be run.
Resources provided
- A worksheet outlining the various possible related tasks. (available on the activity page)
- A number of short videos are available from the activity page to demonstrate the different investigations.
- There is a virtual version of the game, also linked from the activity page.
Resources needed
In addition to the above the following items are desirable;
- Access to computers for the virtual 3D version and the videos.
- Some counters or cubes and paper for the game boards so students can play.
- A real 3D version would be great. (I made the one in the picture above with some old cardboard, some sticks and some blue tack in about 15 minutes.)
Time needs
This task can take anything from an hour to a week and includes an opportunity for students to write a full report on their investigation. Time spent here really depends on the goals of the teacher and the constraints on the class.
Starting and finishing
- I have tried starting this activity in lots of different ways but always find that the 3D case generates the most interest and discussion straight away. 'How many ways are there if getting 3 in a row in a 3 x 3 x 3 playing cube?' and so on.
- It is also an idea to present three or four possible staring points and invite the students to choose their own.
- Once interest is established, it becomes important to ensure students focus on a particular challenge.
- I am wary of the simple case here where 2n + 2 sums the possibilities as it often prompts students to ask why its necessary to use algebra to express this generality when it is obvious without? Of course there are answers to those questions but it sometimes pays to skip to a situation that avoids those questions in the beginning.
- Of course, this activity can be extended and extended. Consider an n x m x p playing cuboid with q in a row to win. How many ways are there?
Records
I suggest a written report is a valuable thing to do here and provides an excellent record of the activity. If teachers can collect and publish examples of really good reports then these records can benefit all students as well.
What to expect
- It can be difficult to get students to define clearly the limiting conditions of their projects. For example, one investigation could be a 'n x n x n playing cube with n in a row to win'. 'n x n x n playing cube with 3 in a row to win' is a different investigation with different generalisations. I think its important to establish these limiting conditions to keep work focussed and in the realms of possibility! Its an equally good opportunity to discuss the very notion of limiting conditions.
- As mentioned above, if you offer lots of starting points then it can be hard to get students to focus on just one of them.
- Expect a good deal of discussion and debate as students refine and test their ideas out loud.
- Students often find it difficult to show what they are saying out loud in written words and diagrams.
